3 4i In Trigonometric Form
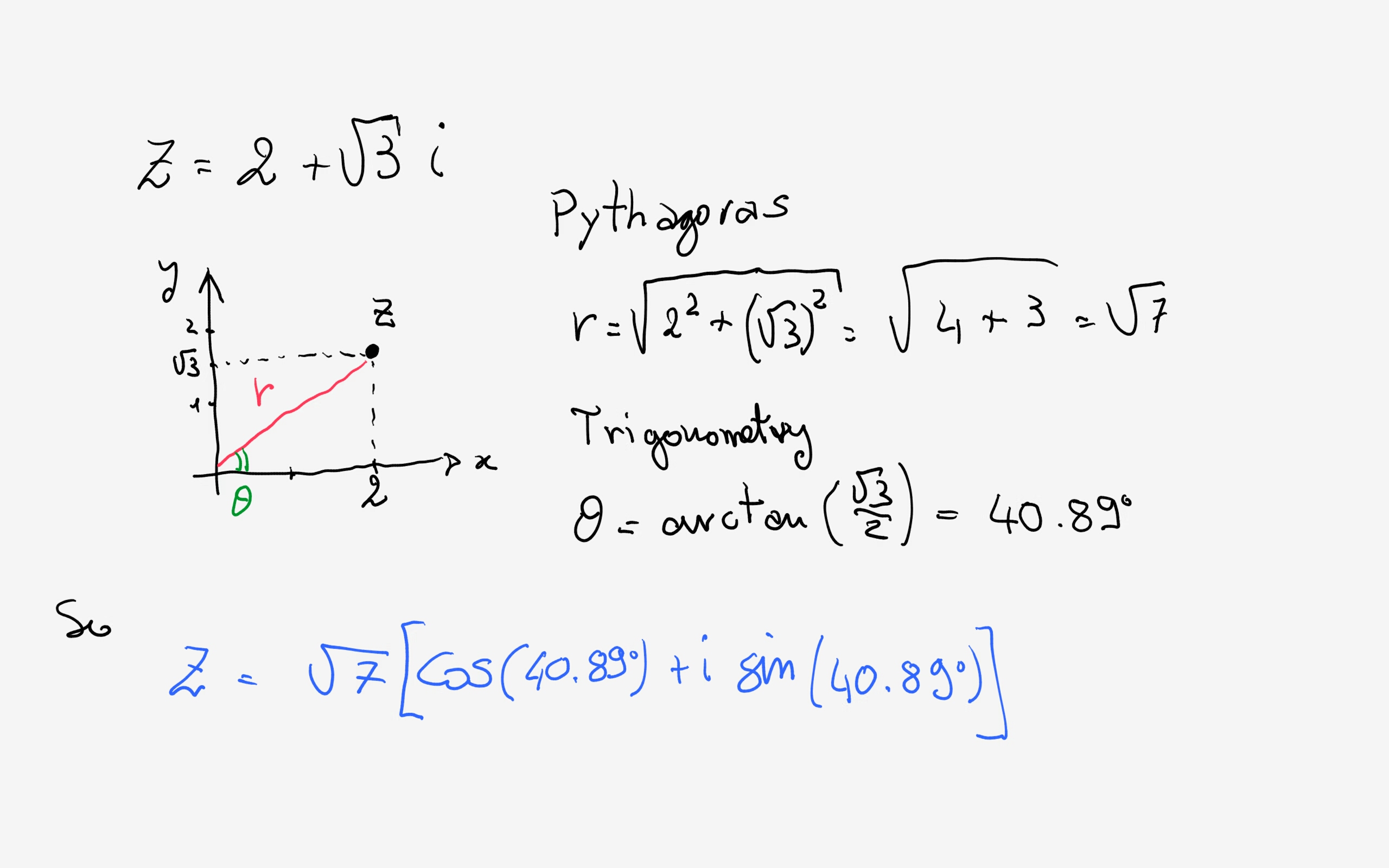
3 4i in trigonometric form - Z = 3+4 i angle notation (phasor): Write the complex number 3 + 4i in trigonometric form r(cos theta + i sin theta), with theta in the interval [0 degree, 360 degree) this problem has been solved! Web 3+4i deg rad auto rectangular form (standard form): (b) use the identity ia ib e e eila+b) to show. Web please subscribe here, thank you!!! The polar form of a complex number z = a + bi is z = r (cos θ + i sin θ). Let's say z = −3 +4i. |z| = √( − 3)2 + 42 = √25 = 5. In order to have the trigonometric form of this complex number, we first need its module. Z = 5 ∠ 53°7'48″ polar form: Web finally, on substituting the modulus and the argument into the standard trigonometric form $z=r\left( \cos \theta +i\sin \theta \right)$, we will obtain the trigonometric form of the. Web the trigonometric form is = 5(cos( − 0.93) +isin( −0.93)) explanation: So, first find the absolute value of. Any complex number z = a +ib can be converted to the polar form z = |z|(cosθ+ isinθ) where,. Z = 5 × (cos 53°7'48″ + i sin 53°7'48″) exponential form:
Z = 3 + 4i Complex Number to Polar Form YouTube
(b) use the identity ia ib e e eila+b) to show. Any complex number z = a +ib can be converted to the polar form z = |z|(cosθ+ isinθ) where,. Web 3+4i deg rad auto rectangular form (standard form): Web finally, on substituting the modulus and the argument into the standard trigonometric form $z=r\left( \cos \theta +i\sin \theta \right)$, we will obtain the trigonometric form of the. So, first find the absolute value of.
Solved Write the complex number 3 + 4i in trigonometric form
Web finally, on substituting the modulus and the argument into the standard trigonometric form $z=r\left( \cos \theta +i\sin \theta \right)$, we will obtain the trigonometric form of the. Web the trigonometric form is = 5(cos( − 0.93) +isin( −0.93)) explanation: Z = 5 ∠ 53°7'48″ polar form: Let's say z = −3 +4i. The polar form of a complex number z = a + bi is z = r (cos θ + i sin θ).
Write the Complex Number in Trigonometric (Polar) Form 4sqrt(3) + 4i YouTube
(b) use the identity ia ib e e eila+b) to show. Z = 3+4 i angle notation (phasor): Z = 5 × (cos 53°7'48″ + i sin 53°7'48″) exponential form: In order to have the trigonometric form of this complex number, we first need its module. Let's say z = −3 +4i.
How to find the two square roots of 34i Quora
Write the complex number 3 + 4i in trigonometric form r(cos theta + i sin theta), with theta in the interval [0 degree, 360 degree) this problem has been solved! Web the trigonometric form is = 5(cos( − 0.93) +isin( −0.93)) explanation: |z| = √( − 3)2 + 42 = √25 = 5. Any complex number z = a +ib can be converted to the polar form z = |z|(cosθ+ isinθ) where,. Let's say z = −3 +4i.
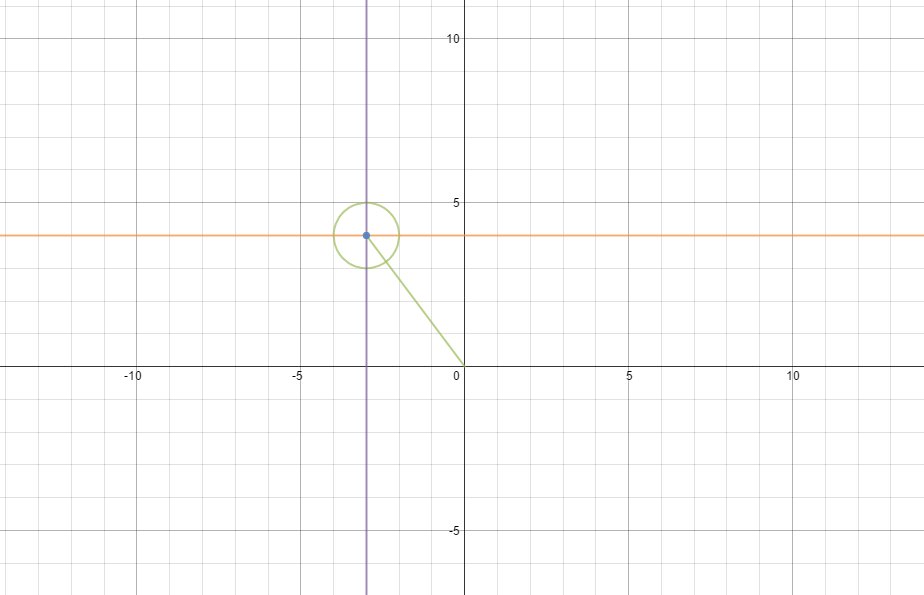
How do you graph the point3+4i on a complex plane? Socratic
In order to have the trigonometric form of this complex number, we first need its module. Web 3+4i deg rad auto rectangular form (standard form): Z = 5 ∠ 53°7'48″ polar form: Web please subscribe here, thank you!!! Web finally, on substituting the modulus and the argument into the standard trigonometric form $z=r\left( \cos \theta +i\sin \theta \right)$, we will obtain the trigonometric form of the.
How do I graph the complex number 3+4i in the complex plane? Socratic
Write the complex number 3 + 4i in trigonometric form r(cos theta + i sin theta), with theta in the interval [0 degree, 360 degree) this problem has been solved! Z = 5 × (cos 53°7'48″ + i sin 53°7'48″) exponential form: So, first find the absolute value of. Web finally, on substituting the modulus and the argument into the standard trigonometric form $z=r\left( \cos \theta +i\sin \theta \right)$, we will obtain the trigonometric form of the. Web please subscribe here, thank you!!!
How to find the two square roots of 34i Quora
Any complex number z = a +ib can be converted to the polar form z = |z|(cosθ+ isinθ) where,. The polar form of a complex number z = a + bi is z = r (cos θ + i sin θ). |z| = √( − 3)2 + 42 = √25 = 5. Z = 5 × (cos 53°7'48″ + i sin 53°7'48″) exponential form: Let's say z = −3 +4i.
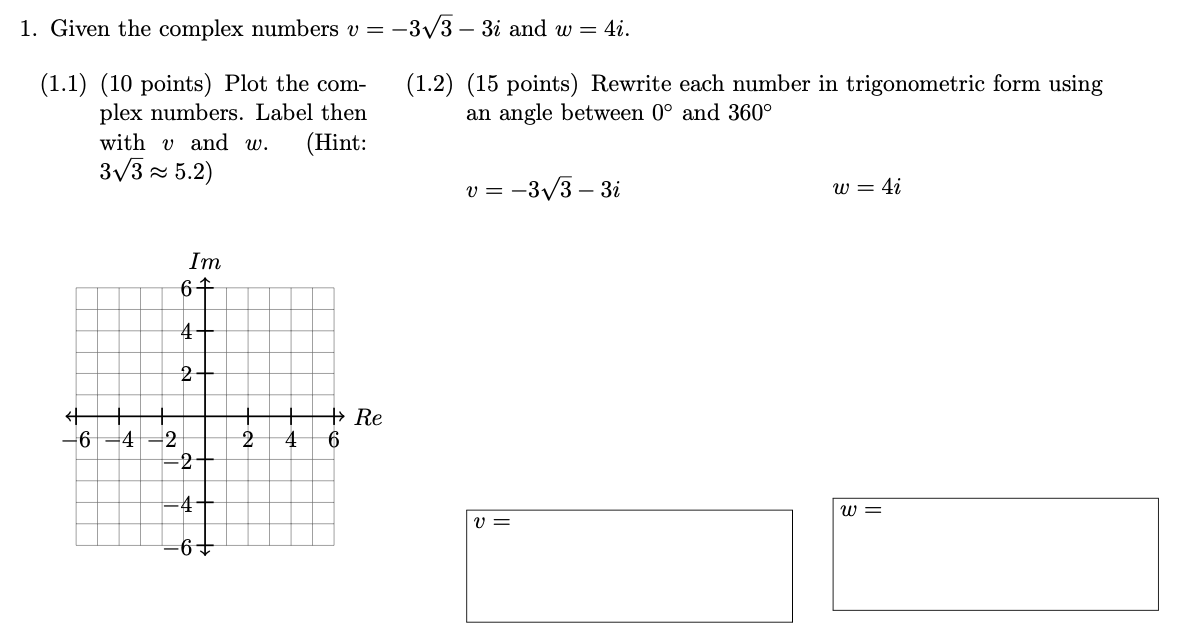
How do you express the complex number in trigonometric form 2+(sqrt 3)i? Socratic
Let's say z = −3 +4i. Z = 5 ∠ 53°7'48″ polar form: |z| = √( − 3)2 + 42 = √25 = 5. The polar form of a complex number z = a + bi is z = r (cos θ + i sin θ). (b) use the identity ia ib e e eila+b) to show.
Trigonometry Recent Questions
Web please subscribe here, thank you!!! Any complex number z = a +ib can be converted to the polar form z = |z|(cosθ+ isinθ) where,. Web finally, on substituting the modulus and the argument into the standard trigonometric form $z=r\left( \cos \theta +i\sin \theta \right)$, we will obtain the trigonometric form of the. In order to have the trigonometric form of this complex number, we first need its module. Write the complex number 3 + 4i in trigonometric form r(cos theta + i sin theta), with theta in the interval [0 degree, 360 degree) this problem has been solved!
How do I use graphing in the complex plane to subtract 3+4i from 2+2i? Socratic
|z| = √( − 3)2 + 42 = √25 = 5. Web finally, on substituting the modulus and the argument into the standard trigonometric form $z=r\left( \cos \theta +i\sin \theta \right)$, we will obtain the trigonometric form of the. Let's say z = −3 +4i. In order to have the trigonometric form of this complex number, we first need its module. Any complex number z = a +ib can be converted to the polar form z = |z|(cosθ+ isinθ) where,.
So, first find the absolute value of. Web please subscribe here, thank you!!! Any complex number z = a +ib can be converted to the polar form z = |z|(cosθ+ isinθ) where,. Web finally, on substituting the modulus and the argument into the standard trigonometric form $z=r\left( \cos \theta +i\sin \theta \right)$, we will obtain the trigonometric form of the. The polar form of a complex number z = a + bi is z = r (cos θ + i sin θ). (b) use the identity ia ib e e eila+b) to show. Z = 5 × (cos 53°7'48″ + i sin 53°7'48″) exponential form: Z = 5 ∠ 53°7'48″ polar form: |z| = √( − 3)2 + 42 = √25 = 5. In order to have the trigonometric form of this complex number, we first need its module.